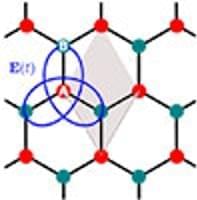
Electrons in two-dimensional hexagonal materials have an extra degree of freedom, the valley pseudospin, that can be used to encode and process quantum information. Valley-selective excitations, governed by the circularly polarized light resonant with the material’s bandgap, are the foundation of valleytronics. It is often assumed that achieving valley selective excitation in pristine graphene with all-optical means is not possible due to the inversion symmetry of the system. Here, we demonstrate that both valley-selective excitation and valley-selective high-harmonic generation can be achieved in pristine graphene by using a combination of two counter-rotating circularly polarized fields, the fundamental and its second harmonic. Controlling the relative phase between the two colors allows us to select the valleys where the electron–hole pairs and higher-order harmonics are generated. We also describe an all-optical method for measuring valley polarization in graphene with a weak probe pulse. This work offers a robust recipe to write and read valley-selective electron excitations in materials with zero bandgap and zero Berry curvature.