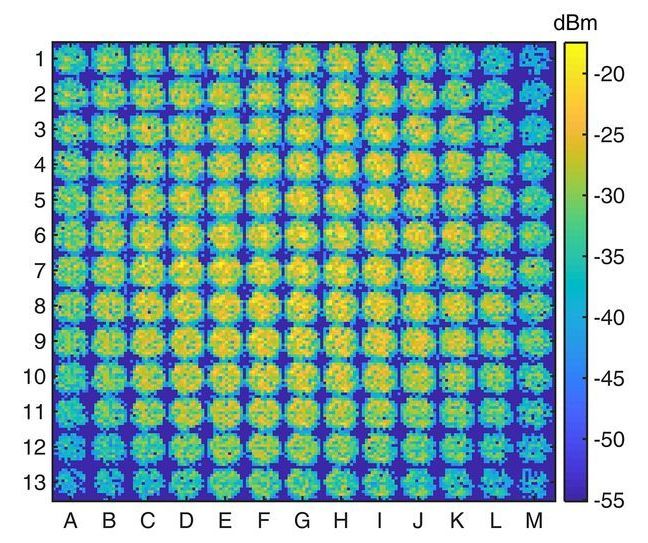
Using the same technology that allows high-frequency signals to travel on regular phone lines, researchers tested sending extremely high-frequency, 200 GHz signals through a pair of copper wires. The result is a link that can move data at rates of terabits per second, significantly faster than currently available channels.
While the technology to disentangle multiple, parallel signals moving through a channel already exists, thanks to signal processing methods developed by John Cioffi, the inventor of digital subscriber lines, or DSL, questions remained related to the effectiveness of implementing these ideas at higher frequencies.
To test the transmission of data at higher frequencies, authors of a paper published this week in Applied Physics Letters used experimental measurements and mathematical modeling to characterize the input and output signals in a waveguide.