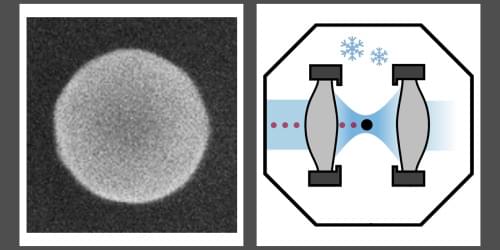
A team of researchers has discovered how a little-known type of symmetry in quantum materials, called nonsymmorphic symmetry, governs the way these materials interact with intense laser light.


In 2018, results from the MiniBooNE neutrino experiment suggested the exciting possibility that low-energy muon neutrinos quantum-mechanically flip into electron neutrinos more frequently than predicted by the standard model of particle physics. Theorists have sought to explain this anomaly, known as the low-energy excess (LEE), by invoking beyond-standard-model explanations such as the existence of new flavors of neutrinos (see Viewpoint: The Plot Thickens for a Fourth Neutrino). However, there was always the possibility that photon emission attributed to electron-neutrino interactions had been caused by other processes. Now, an analysis of five years of data from MicroBooNE, a follow-up experiment with a different design, has effectively ruled out the electron-neutrino-based interpretation of the LEE [1].
MiniBooNE operated by observing the Cherenkov radiation from fast-moving charged particles generated by neutrino–nucleus interactions in the 800 tonnes of mineral oil that constituted the detector’s sensitive volume. But the experiment could not easily exclude photons from other sources. MicroBooNE has a smaller sensitive volume composed of liquid argon, but it can reconstruct charged particles’ trajectories and energies precisely, allowing it to identify photon origins more reliably. As well as taking advantage of this intrinsic selectivity, the MicroBooNE team took elaborate steps to reduce all sources of uncertainty, both instrumental and theoretical.
The resulting high-quality data show good agreement with the standard-model predictions. By comparing these results with those from MiniBooNE, the researchers were able to exclude the electron-neutrino-based explanation for the apparent LEE at a confidence level of over 99%. While this conclusion might be disappointing for some, it compels scientists to look for new explanations for the MiniBooNE anomaly, the cause of which is still unknown.

To build a large-scale quantum computer that works, scientists and engineers need to overcome the spontaneous errors that quantum bits, or qubits, create as they operate.
Scientists encode these building blocks of quantum information to suppress errors in other qubits so that a minority can operate in a way that produces useful outcomes.
As the number of useful (or logical) qubits grows, the number of physical qubits required grows even further. As this scales up, the sheer number of qubits needed to create a useful quantum machine becomes an engineering nightmare.
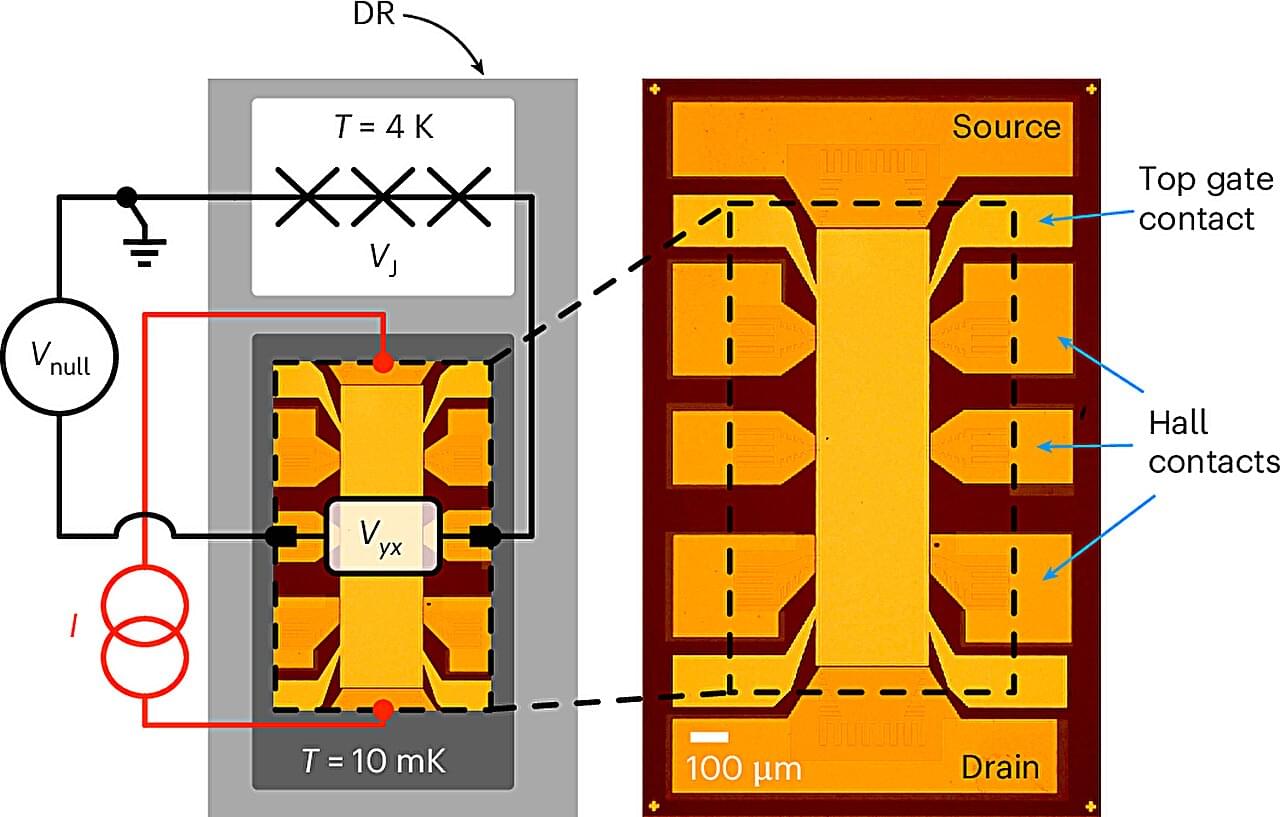
A team of scientists has revealed how a single quantum device can accurately measure the three fundamental units of electricity—the ampere (unit of electrical current), the volt (unit of electrical potential) and the ohm (unit of electrical resistance). This is a significant breakthrough because until now, no single instrument could measure all three primary electrical units in one practical system. It means that making electrical measurements could be more precise and reduce the potential for human error.

At first glance, biology and quantum technology seem incompatible. Living systems operate in warm, noisy environments full of constant motion, while quantum technology typically requires extreme isolation and temperatures near absolute zero to function.
But quantum mechanics is the foundation of everything, including in biological molecules. Now, researchers at the University of Chicago Pritzker School of Molecular Engineering (UChicago PME) have turned a protein found in living cells into a functioning quantum bit (qubit), the foundation of quantum technologies. The protein qubit can be used as a quantum sensor capable of detecting minute changes and ultimately offering unprecedented insight into biological processes.
“Rather than taking a conventional quantum sensor and trying to camouflage it to enter a biological system, we wanted to explore the idea of using a biological system itself and developing it into a qubit,” said David Awschalom, co-principal investigator of the project, Liew Family Professor of Molecular Engineering at UChicago PME and director of the Chicago Quantum Exchange (CQE). “Harnessing nature to create powerful families of quantum sensors—that’s the new direction here.”

Everyone who ever took a photo knows the problem: if you want a detailed image, you need a lot of light. In microscopy, however, too much light is often harmful to the sample—for example, when imaging sensitive biological structures or investigating quantum particles. The aim is therefore to gather as much information as possible about the object under observation with a given amount of light.
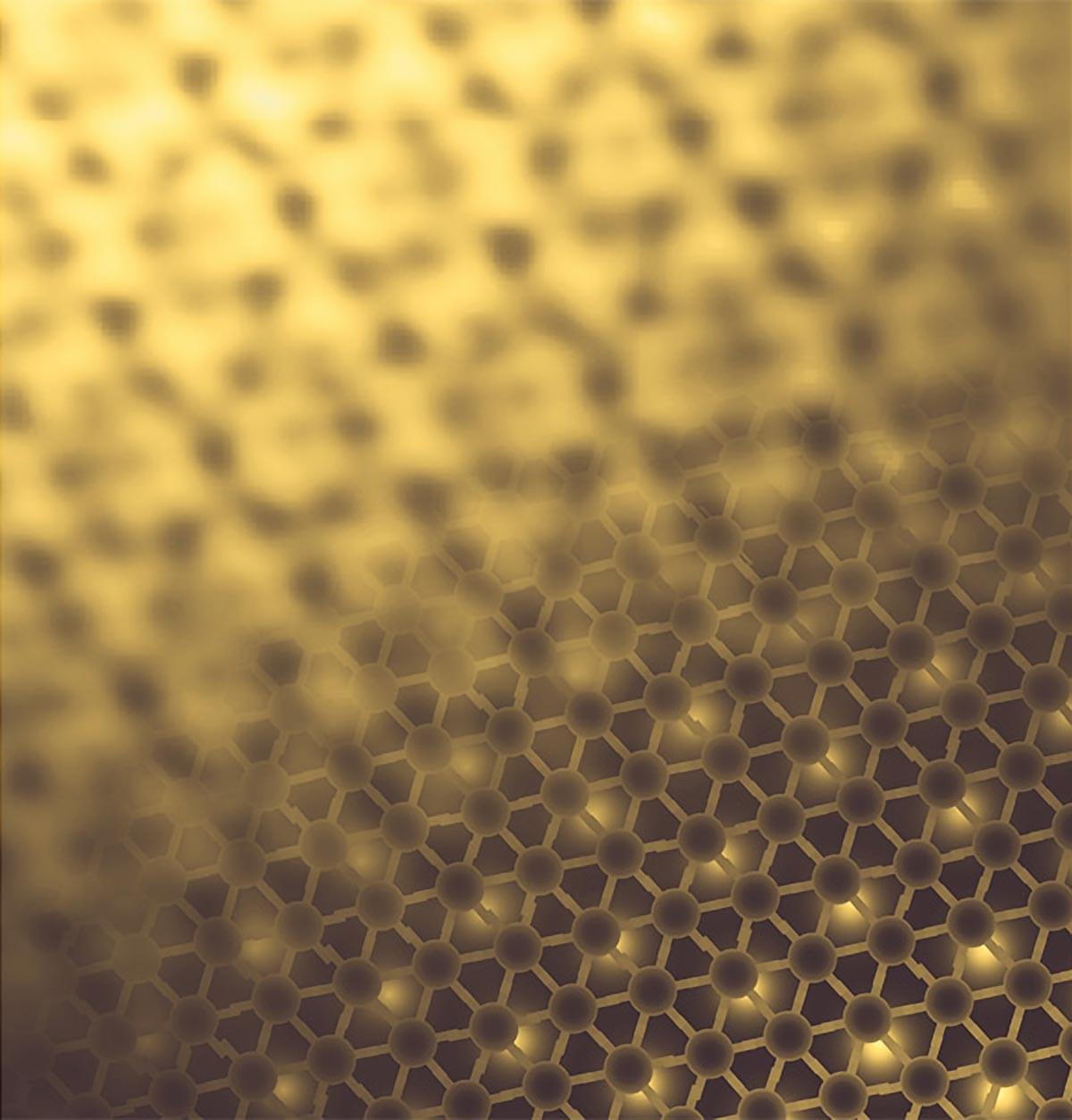
Scientists at Rutgers University-New Brunswick have identified a new type of material known as intercrystals, which display unusual electronic behaviors that may help shape future technologies.
According to the research team, intercrystals demonstrate electronic characteristics not previously observed, opening the door to progress in areas such as advanced electronic devices, quantum computing.
Quantum computers exploit superposition and entanglement to solve complex problems that are intractable for traditional computers.