Lifeboat Foundation

The popularity of remote food delivery skyrocketed during the COVID-19 pandemic, and the trend has continued to help businesses thrive years later. Unfortunately, some of the robotic delivery vehicles are taking a beating, with several viral videos showing people kicking the autonomous bots over and even stealing the products inside.
KTLA 5’s Rachel Menitoff reports. (Aug. 7, 2023)

KTLA 5 News — Keeping Southern Californians informed since 1947.

“The invention, which cooks cylinder-shaped pizzas and other foods, recently moved on to the final phase of NASA’s Deep Space Food Challenge.”

The invention, which cooks cylinder-shaped pizzas and other foods, is now being adjusted for zero-gravity with the help of BioServe Space Technologies.

Wow what a great story.

https://www.facebook.com/groups/weirdbizarreandbeautiful/per…tid=Nif5oz

Continue reading “Bury an Egg and Banana in Your Garden and Boost Your Plants” »

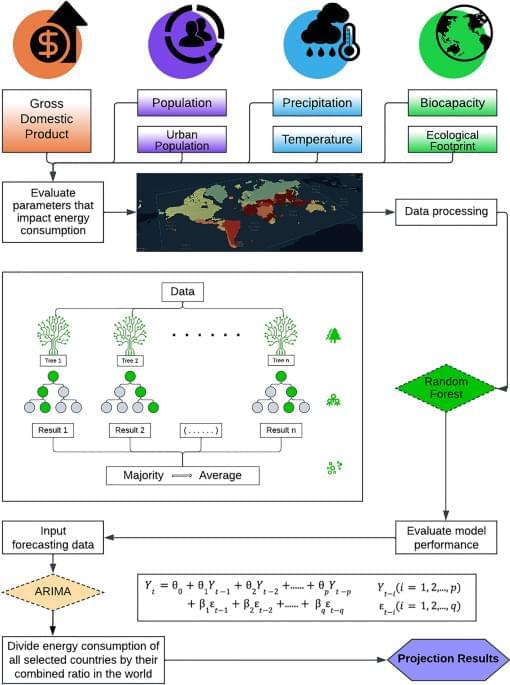
Throughout the history of human civilization, energy has been holding an imperative role in humanity’s progress¹. Especially in the past few centuries, innovations in the harnessing of power have catalyzed humanity’s rapid growth². Energy remains a key driver of human development³, with each revolution in industry and agriculture highlighting human’s reliance on it. Revolution in the eighteenth century was a turning point. The development of steam engines powered by fossil fuels led to significant technological progress⁴. Electricity has then opened new possibilities for the future⁵. Humanity has grown at a compound annual rate of 2.43% from 1965 to 2020, demonstrating our continued increasing demand for and consumption of energy^6,7. However, the pace at which human being can progress as a civilization in the future remains uncertain.

While mankind was establishing its identity in the universe, insatiable human curiosity over the realm of civilization peaked in the 1960s⁸, which led to deeper cogitation of the concept of civilization. Providing that some of the extraterrestrial civilizations are highly likely million years more advanced than mankind, Soviet astrophysicist Nikolai Kardashev proposed a scale to classifies a civilization’s technological development based on its energy consumption⁹, which was later known as the Kardashev Scale. The scale initially categorized civilizations into three types. Type 1 is known as the planetary civilization, which features the capability of harnessing and utilizing all forms of energies that can be reached on the host planet, such as wind, solar, and geothermal power; Type 2 and 3, known as the stellar and galactic civilizations, respectively, are capable of extracting and utilizing all energy created by their respective systems⁹. Yet, such a scale proved lackluster in the quantitative presentation of the civilization types. Subsequently, Carl Sagan furthered the Kardashev Scale with data extrapolation, and proposed a continuous function quantifying the Kardashev Scale in index K¹⁰

$$K=\frac{.

A new discovery by the Polytechnic University of Milan opens up new perspectives in the field of sustainable chemical synthesis, promoting innovative solutions that allow chemicals to be created in a more efficient and environmentally friendly way. The findings were recently published in the journal Nature Synthesis.

Using the innovative technique of dispersing isolated atoms on carbon nitride supports, the team developed a catalyst that is more active and selective in esterification reactions. This is an important reaction in which carboxylic acids and bromides are combined to form products used in the manufacture of medicines, food additives, and polymers.

The revolutionary feature of this new catalyst is that it reduces the use of rare metals, a significant step towards conserving critical resources and making processes more sustainable. In addition, the catalyst can be activated by sunlight, eliminating the need for energy-intensive methods. This discovery holds enormous potential in reducing dependence on finite resources and lowering the environmental impact of catalytic processes.

Pork ribs for vegans.

Exclusive: Company says some may see the bones as ‘ideological provocation’ but motivation was avoiding waste.

An ancient relative of modern seals—known as Potamotherium valletoni—that had an otter-like appearance and lived over 23 million years ago likely used its whiskers to forage for food and explore underwater environments, according to a new study in Communications Biology. The findings provide further insight into how ancient seals transitioned from life on land to life underwater.

Although modern seals live in marine environments and use their whiskers to locate food by sensing vibrations in the water, ancient seal relatives mostly lived on land or in freshwater environments. Some species used their forelimbs to explore their surroundings. Prior to this study, it was unclear when seals and their relatives began using their whiskers to forage.

Alexandra van der Geer and colleagues investigated the evolution of whisker-foraging behaviors in seals by comparing the brain structures of Potamotherium with those of six extinct and 31 living meat-eating mammals, including mustelids, bears, and seal relatives. Brain structures were inferred from casts taken from the inside of skulls.

Since this book is about what I consider intellectual subject matter, I think it’s relevant to keep brains in top shape and thought it would be important to share this. You probably know about this sort of thing but I didn’t know the specific nutrients needed and what was lacking in people with Alzheimer’s. Best wishes.

Alzheimer’s disease is a progressive neurodegenerative disease estimated to affect 6 million Americans and 33 million people worldwide. Large numbers of those affected have not yet been diagnosed.

A new study published in the Journal of Alzheimer’s Disease by a Virginia Tech Carilion School of Medicine faculty member shows that brain levels of dietary lutein, zeaxanthin, lycopene, and vitamin E in those with Alzheimer’s disease are half those in normal brains. Higher dietary levels of lutein and zeaxanthin have been strongly linked to better cognitive functions and lower risk for dementia or Alzheimer’s disease.

Continue reading “Brains with Alzheimer’s disease have subnormal levels of important dietary antioxidants” »

New research indicates that Australia and New Zealand are the two best places on Earth to survive a nuclear war. The recently published set of calculations don’t focus on blast-related deaths or even deaths caused by radiation fall-out, which most estimates say would number in the hundreds of millions, but instead look at how a nuclear winter caused by nuclear bomb explosions would affect food supplies, potentially leading to the starvation of billions.

Nuclear War Simulations Performed For Decades

Since the first atomic bombs were dropped on the Japanese cities of Hiroshima and Nagasaki in 1945, effectively spelling the end of World War II, war game theorists have looked at a myriad of simulations to determine the potential effects of a full-blown nuclear battle. Many simulations look at the potentially hundreds of millions that would likely die in the initial blasts, while others have tried to model the slower but equally as deadly body count from radiation sickness.